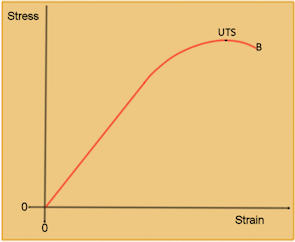
Stress and strain

Stress

Forces on a component, e.g. A bar, are often referred to as loads.
The load that a particular component can withstand will depend on the dimensions of the component as well as the material it is made from; in particular its cross sectional area when subjected to direct forces.

For example if the cross sectional area is doubled then the load that the component can withstand will be doubled.
Therefore what is important is the intensity of loading i.e. The load per unit area, and this is given the name direct stress. Often the word ‘direct’ is omitted.

Hence

Stress = Force
Area

Or in symbols

σ = F
      A

Example 1: A rectangular bar of dimensions 5mm breadth x 20 mm depth, supports a load of 4900 N. Determine the stress in the bar.

Known values

F = 4900 N and A = 5 x 20 = 100mm²

Then σ = 4900 = 49 N/mm²
                100

Ultimate tenisle strength and breaking stress

A stress large enough to break the material is known as the breaking stess.

Stress is literally pulling apart the material material on an atomic scale which is why the material eventually breaks apart completely if enough stress is applied. This breaking point is labelled as B in the graph above and UTS or the ultimate tensile strength shown on the graph is just that, the maximum stress that the material can withstand, these are very important factors to consider when designing.

Strain

In addition to controlling the stress produced in a component, the dimensional changes also require consideration.
In the case of a bar loaded in tension the extension of the bar depends upon its total length. The bar is said to be strained and the strain is defined as the fractional change in the length of the bar.

Hence

Strain = Change in length
             Original length

Note strain does not have any units.

Example 2: A wire of length 2 m, extends by 0.25 mm when acted upon by a tensile force. Determine the strain in the wire.

From ε = x
               l

ε = 0.25
   2 x 10³

= 1.25 x 10¯⁴

< < < Back :