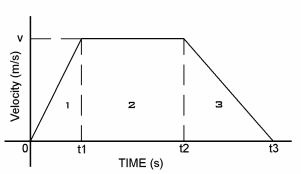
Kinematics

Velocity - Time Graph

Sometimes it is useful in solving problems in kinematics if a velcoity - time graph is drawn first.

For example, if a body accelerates uniformly from rest, then travels at a constant velocity v before retarding uniformly to rest again the graph (fig:1)will take the folowing shape:

Fig:1

Note: The section numbers below relate to those of the graph above.

Section 1

acceleration is given by:  

Therefore                          (since u = 0)

The slope of the graph = acceleration

Simimlarly for distance travelled:

Therefore     

Distance travelled is given by the area under the graph for section 1.

Section 2

Distance travelled: which again is the area under the graph for this section.

Section 3

As in sections 1. and 2. the distance travelled is given by the area under the graph for this
section, i.e.

The acceleration is given by: i.e. the slope of the graph.

Note that this time the slope of the graph and acceleration are negative because the body is slowing down.